From Coffee to Mathematics
August 10, 2023Duminil-Copin is the guest speaker at the ETH Global Lecture Series From Coffee to Mathematics, and he’s determined to make sure all of us – from ETH students to the general public in attendance – understand what he’s talking about.

Duminil-Copin is introduced by Catherine Robert, from the French Embassy in Bern. Professor of mathematics at the Institut des Hautes Etudes Scientifiques in Bures-Sur-Yvette, he is the winner of the Fields Medal, the top prize for mathematics that is handed out at the International Congress of the International Mathematical Union every four years. We are reminded of this fact later in his presentation when he uses images of his Fields Medal to illustrate flipping a coin.
The professor is here to tell us about his work on phase transitioning. His method for catching and keeping our attention lies in using simple comparisons – like slowly turning a game of Hex into a mathematical Ising model of magnetism – served with large helpings of humour.
Duminil-Copin tells us that the job of a mathematician in layman’s terms is finding links that don’t seem connected. Such as: How is the game of Hex connected to coffee, and how are magnets connected to melting polar ice caps? He begins with a game called Hex, which is very popular with mathematicians.
If you are also not familiar with Hex, here are the basics: it is a two-player strategic game, played on a board in the shape of a rhombus made up of 11×11 hexagonal spaces, for example. Each player gets assigned a colour and opposite sides of the board – one plays horizontally, the other vertically. They alternate trying to connect spaces by placing their colour into them until one player creates a chain of adjacent spaces, successfully connecting their sides together. Because the board is arranged symmetrically, there cannot be a draw, there can only be one winner.
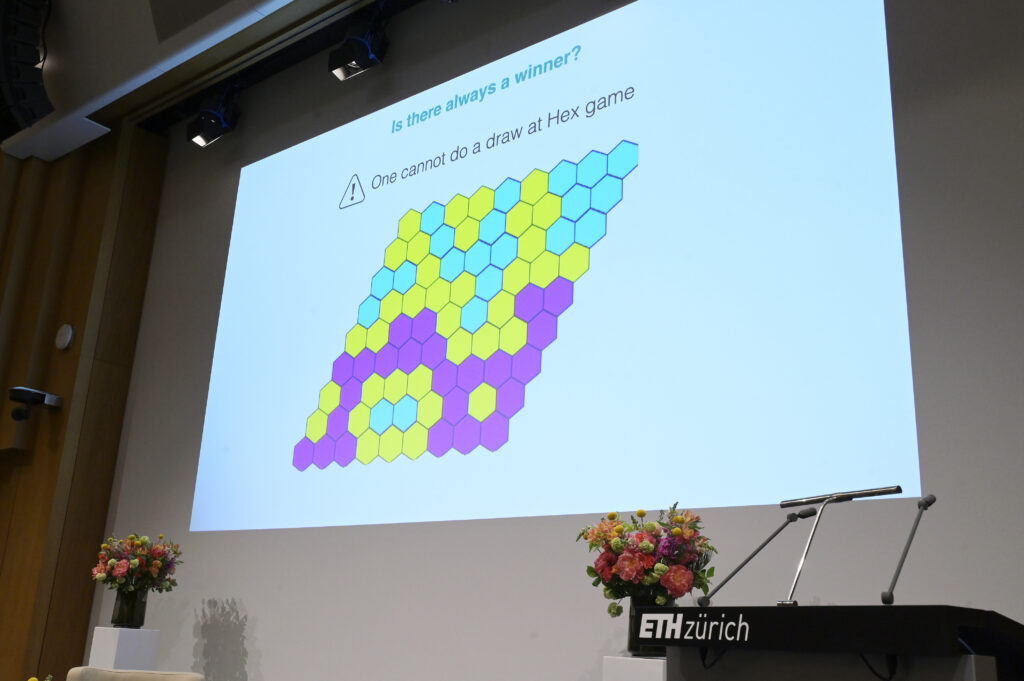
Duminil-Copin explains that the more symmetry an object has, the easier it is to manipulate. The symmetry of conformal maps, where the right angles of latitude and longitude remain in place even when the map is expanded, translated or rotated are is found on globes or Mercator maps. If you can change the Hex board into a conformal map, then the probability of winning is the same.
This image of one side winning is an illustration of the theory of percolation, in which connections create a network of clusters for a type of phase transition that is geometric. “Conformal symmetry allows you to understand a lot of things,” says Duminil-Copin, which is why mathematicians like symmetry.
So the Hex game is an example of percolation. Especially older generations, Duminil-Copin says, will be familiar with the coffee percolator, which solves the first puzzle linking Hex to coffee – with the hot water pushing up through the coffee to the top.

Our second puzzle is the connection between magnets and melting polar ice caps. Duminil-Copin takes us to the Arctic, where percolation, or the porosity of material, is having an effect on the planet. When the sun melts the ice on the surface, the resulting water percolates down, making small holes in the ice. The water in those holes then refreezes and expands, taking up more space as ice. This creates more holes, continuing the process until the ice breaks up.
How does this connect to the magnets? During his studies, Duminil-Copin explains, he looked at how magnets react to heat. And created a model proving how at a certain temperature – the Curie point, named after Pierre Curie – the steel loses its magnetism. This type of phase transition – a brutal change of behaviour in a system as parameters vary – is also reflected in the melding ice caps.
Duminil-Copin tries to understand this type of phase transition through mathematical modelling like the Ising model of magnetism, which looks a lot like the Hex game. He explains that by studying this model, you can do a lot of things, like using the percolation theory to prove that the phase transition of a 3D Ising model is continuous.
As he shows the stages from game to model, I suddenly found myself understanding things that would have given me a headache just 45 minutes before.

The importance of knowing how mathematicians like Duminil-Copin work is made clear: “We live in a world where we want to understand things immediately. And this is very far from what we do in math and what sciences do in general. And (…) one of the reasons (…) this discussion between scientists and the public (was) difficult during the pandemic is that the people actually discovered research. They were only (faced with) science before, the result of the process. The process of research (results in) understanding something difficult through mistakes and (that’s) something that takes time.”
After Duminil-Copin’s lecture, the questions from the audience come mainly from ETH students, so things get complicated really quickly. But one stands out:
“How do we know that percolation is a good model for porosity of material?”
“First, I’m going to give you the non-politically correct answer, which is that I don’t care so much,” Duminil-Copin jokes. “It’s (just) a beautiful mathematical model, so that is my main motivation,” he adds. And laughs.
Watch the full recording:
Find out more about our ETH Global Lecture Series here.





